But to clarify and deepen those observations we need something to know about DYNAMICAL SYSTEMS. Of course that means : natural dynamical systems, i.e. concrete, material, physical or biological systems. We shall discuss them. But we must emphasize that those natural systems are generally very complex, and only partially understood, especially the biological ones. Therefore we shall concentrate our expositions on abstract dynamical systems in the form of computer simulations. Which in fact means that when we discuss real dynamical systems, we are inspired by those abstract systems. Such systems are fully defined, and because of that better understood. Maybe they can supply us with the proper concepts needed for a revised Substance-Accident Metaphysics. We must thereby keep in mind, however, that those simulations are relatively simple and are not able to supply all the concepts needed.
By means of the study of dynamical systems we hope to find out more about the status of Substance, Accident, Essence, Individual, the per se and the per accidens. Maybe we can do this by means of conceps like Dynamical Law, System State, Initial Condition, System elements, Attractors, Phase-portraits, Attractor Basin Fields, Dynamical Stability, and so on, which in fact means the following : an ontological interpretation of those concepts.
"Ontology " means here : The study of Being as such, the way of being of an individual thing, the status of being of the Essence, the status of being of the Universal, the status and way of being of properties in relation to Substance, the status of a process in terms of being or becoming, etc.
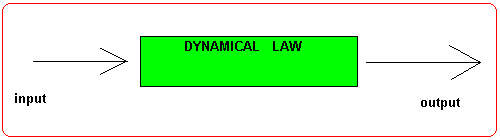
A DYNAMICAL SYSTEM is a process that generates a sequence of states (stadia) on the basis of a certain dynamical law.
Such a dynamical law, together with a starting-state (initial condition) is already the whole dynamical system.
When the system states together form a continuous sequence, the dynamical law will have the form of (i.e. will be described with) one, or a set of, differential equations, which describe, and dictate as a law, the changes of one or more quantities in time (and in space), and so (describe and dictate) the continuous sequence of states.
When, on the other hand, those states together form a discrete sequence, then the dynamical law will express a constant relation between (every time) the present state and the next state, and this relation is of such a nature that no infinitesimals are involved (in other words, the differences between successive states are nowhere infinitely small).
Every process state (system state) is a (certain) configuration of, ultimately, system elements , which (configuration) is generally different with each successive process state. The dynamical law according to which those configurational changes proceed, is immanent in the (properties of the) system elements (For where else should it be seated?). The changes in the element configuration, and so also the (implied) succession of process states, is the effect of (i.e. is caused by) interactions between system elements (In such a process it is possible that some system elements disintegrate to other elements or form compounds with other elements, and then those products will interact with one another). These interactions are the concrete embodiment of the dynamical law in action.
The changes of configuration could be such, that we can (after the fact) speak of SELF-ORGANIZATION of the system elements towards a coherent stable PATTERN. This pattern can be a final configuration of the system elements, to which the system clings, i.e. never leaving this configuration anymore. But the above mentioned pattern can also be a dynamical pattern, which also is coherent in itself, but which moreover alternates in a regular and coherent way.
Both cases of self-organisation can be interpreted as the formation of an organized whole, and this we will call a Totality (in all cases of real systems, that means a Uniform Being ), especially when the generated (dynamic or otherwise) pattern shows an intrinsic delimitation (a boundary) with an environment. When this all happens we speak of a Totality-generating dynamical system.
An ontological interpretation of a macroscopic Totality (a uniform thing) in terms of dynamical systems will proceed along the following lines :
We will first of all presuppose (the presence of) a Totality-generating dynamical system.
The process stadia of such a system now imply the corresponding process
stadia of the Totality. A process stadium of a Totality is the ' Here-and-now Individual ' -- we can call this also : the Semaphoront -- while, all those stadia taken together, form the ' Historical Individual '.
Generation of a Totality means that the system elements, or a part thereof, together form a coherent whole, a totality-resultant of the dynamical system, coherent, either in space, or in time, or in space and time, and having an intrinsic boundary with an environment. So not just any (arbitrarily chosen) process (dynamical system) generates a Totality. Especially for abstract ' dynamical ' systems -- which are moreover just simulations of dynamical systems -- applies the following : They cannot supply all the revised metaphysical concepts, needed for the establishment of a revised Substance-Accident Metaphysics. The dynamical systems that are involved in the generation of full-fledged Totalities thus have some special properties.
Every process stadium is a certain configuration of system elements and can be considered as an initial state , meaning that the system will be observed from that state onwards.
The real , actual (= ' historical ') initial state also is a configuration of system elements, but as configuration it originates from outside the system. It could even be a configuration which in principle cannot be generated by the system from other configurations (i.e. from other states). Such an actual initial state can also be random (i.e. a random arrangement of elements, or / and random states of the elements themselves). But the system can transform this random configuration, once given, into a (following) PATTERN (= a not-random configuration), and in turn into a next pattern, etc., and so leading to a sequencing of patterned process stadia. In other words the system is then able to organize the constituents into a real pattern. The elements (constituents) are going to take part in (the formation of) a Totality. The sequence of process stadia, taken as a whole, also can be considered as a pattern when it gives a reason to do so. But a real Totality is only formed when such a pattern has a, for the system intrinsic, boundary with an environment.
A local, individual action originating from the environment, thus coming from outside a running dynamical system, can be considered as a perturbation of a current process state. The perturbation then creates, as it were, a new initial state with respect to that process.
The relevant properties of the constituents (the system elements) of that process determine the nature of their interactions. Thus that which determines those interactions as such and such (a way) taking place, is immanent with respect to the constituents. The whole system, and thus the whole process, is further constrained by the general (global) state of the environment and thus by the general nature of physical matter, described by Natural Science in the form of general -- i.e. everywhere operating -- Natural Laws. These global (i.e. operating on a global scale) Laws of Nature are immanent, i.e. inherent in the general properties of physical matter.
The mentioned -- (mentioned) with respect to the taking place of the interaction process -- relevant properties of the system constituents can also in this non-global case -- thus in the case of a special process, taking place somewhere, generating a Totality -- be interpreted as a law, namely the law that is valid for specifically that (type of) dynamical system : The Dynamical Law. This law is, as has been said, immanent in the relevant elements of the system. The pattern, i.e. the arrangement -- at a certain point of time -- of these elements is extrinsic with respect to that Dynamical Law. It even could, as have been said, be an arrangement (configuration of system elements) which cannot even in principle be reached by the system itself from whatever initial condition. Such an unreachable state, which as such can only originate from outside the system (= from outside being imposed on the system), is called a ' Garden of Eden State ' of the system (Theoretical models learn that many systems each for themselves have a large proportion of such Garden of Eden States). Such an unreachable configuration (of system elements) either is a real starting state of the dynamical system (i.e. the system happened to start just with such a configuration), and so is coming from outside the system, or such a state is the result of a perturbation, which took place at some point in time during the running of the system, and so is also coming from outside the system, a perturbation of a process state (situated) higher up in the sequence. Thus by actions from outside, a current process state, itself also being a configuration of system elements, can be changed, resulting in a new, i.e. other, configuration of system elements, which then functions as an initial state with respect to the further history of the process.
So a dynamical system implies a number of types (meanings) of : " outside the system " :
Before we explain these two ways, something must first be said about the status of element :
With " elements coming in from outside the system (and therefore quasi elements) " I mean elements which are not imported by the dynamical system itself, but which, by accident end up within the active domain of the system. If a Totality, or more generally, a pattern (of a higher order than that of the system elements themselves) is being generated within the active medium of the dynamical system, then it is possible that the elements belonging to that active medium, as well as possibly elements coming from without (that active medium), are going to participate in the formation of the Totality (the unified pattern, being generated by the dynamical system). The insertion into that Totality, in this last mentioned case (elements coming in from without), does not happen by virtue of the Dynamical Law of the relevant dynamical system, but is a perturbation from without. The present context is concerned with the effect of elements-coming-from-without on the stability of the dynamical system.
Now we are ready to discuss the above mentioned two ways by which the system tries to maintain itself :
If a given real dynamical system generates a full-fledged Totality, for instance (generating) a crystal from a solution, then this Totality is a Substance (in the metaphysical sense of the term), more specifically, it is a First Substance.
The Essence or (ontological) Second Substance, is the Dynamical Law of such a system.
All the observable properties of such a Totality are generated by the system. These properties are called Accidents (although they do not all have a status of 'generated by accident' ), and will be all kinds of quantitative properties like length, volume and the like, but also qualitative properties like configuration (which can end up as colors, densities and the like).
All these observable entities, the First Substance and its properties, are, as has been said, generated.
Borrowing terms from Genetics, we could say that those generated entities are seated in the 'phenotypical' domain (a domain of being, a way of being), while the corresponding Dynamical Law is seated in the 'genotypical' domain (another domain of being, another way of being). We discriminate between these domains, because the Dynamical Law as such is not observable. It abides in the collection of system elements, i.e. it is dispersed over those elements, without being the same as those elements because it is only dispersed over some (not all) aspects of every system element. Therefore the Dynamical Law is neither a thing, nor a property. It is abstract.
The First Substance and its properties are, on the contrary, concrete and directly observable.
The mentioned accidents belong to that first substance of which they are accidents. Some of them belong to it per se, others only per accidens.
All those accidents together make up the first substance [NOTE 2]. They can only exist as a first substance. They cannot exist on their own, because they are, each for themselves, just a determination of a first substance.
Remark : A different initial condition does not correspond to a different settling pattern in a per se manner. So, often a certain set of different initial conditions exists, each member of which bringing the system to the same settling pattern. But such a set need not be the total set of possible initial conditions with respect to the system.
The total set of states, belonging to, and arranged according to, all possible trajectories, all leading to a certain attractor, say attractor A(1), forms, together with the attractor states themselves, the basin of attraction of the attractor A(1) (analogous to all the rivers that drain a certain area and all end up in the same lake).
The total of all possible basins of attraction belonging to (i.e. corresponding to) a certain dynamical system is called the phase portrait (this term is used in the case of continuous systems) of that system, or the attraction-basin field (term used for discrete systems).
The attraction-basin field represents all possible systemstate-transitions of that dynamical system, and is, in a way, equivalent to the Dynamical Law of that system.
The Dynamical Law is the system law (seated) at a low structural level, while the corresponding attraction-basin field is this same law, but now (seen) from a global structural level.
There exist dynamical systems, for instance abstract Boolean Networks (and their real counterparts), where the dynamical behavior depends on a whole set of dynamical laws, but which nevertheless have only ONE attraction-basin field. It seems reasonable to interpret this attraction-basin field as THE (one) Law of the system, and so also as the Essence of the Totality (when a Totality is indeed generated by the system). But of course the mentioned set of dynamical laws can also be interpreted as THE (one) Dynamical Law and so as the Essence of the (generated) Totality.
Just the set of all possible system states corresponding with a certain dynamical system is called the phase-space (term used for continuous systems) or the state space (term used for discrete systems) of the system. The system thus organizes its state-space into (a relatively small number of) basins of attraction, the attraction-basin field, by establishing all its possible state transitions (which means that the possible states are now related to each other in a specific way). And so the dynamical system ' categorizes ' the state-space and because of that the resulting attraction-basin field can be considered as the ' memory ' of the system, especially when such a system is a Boolean Network. A Boolean Network is a discrete dynamical system with only two-valued variables. Such systems constitute a possible basis for the study of genetic and neural networks (See the Essay on Boolean Systems).
The above given interpretation of the notions Totality, Identity, Essence, Here-and-now Individual ( Semaphoront ), Historical Individual, etc. is inspired by the study of simple abstract models of dynamical systems (in the form of computer simulations), which pretend to represent processes, and aspects thereof, in the Real World, especially those processes which show self-organisation of system elements towards stable coherent patterns. Such are for instance crystallisation processes and ontogenetic processes (the last mentioned are processes relating to the formation of an individual organism).
But we must realize that we, in proceeding along these lines, make use of formidable simplifications of natural real processes (natural real dynamical systems), resulting in such models, i.e. reducing them to such models. This is, according to me, inevitable because the processes in the Real World generally are much too complex and much too strongly interweaved and intertwined with other processes, as to allow directly from them (i.e. them serving as a theoretical point of departure) a definitive ontological interpretation of such Totality-generating processes. The models must be part of the point of departure of such an attempt to an ontological interpretation.
Having studied dynamical systems in general (especially with respect to an ontological interpretation of some concepts) the reader is invited to study a specific example of a dynamical system , in order to make his impressions more lively.